Permutations and combinations

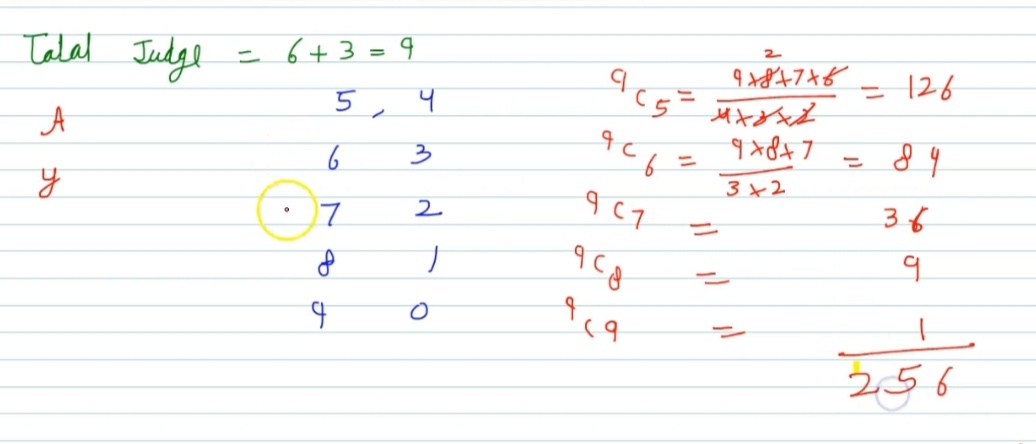
Correct option is D 3 ladies out of 8 can be selected in 8​C3​ ways and 4 gentlemen out of 7 in 7​C4​ ways. Now each way of selecting 3 ladies is associated with each way of selecting 4 gentlemen. Hence, the required number of ways =8​C3​×7​C4​=56×35=1960. We now find the number of committee of 3 ladies and 4 gentlemen in which both Mrs X and Mr Y are member. In this case we can select 2 other ladies from the remaining 7 in 7​C2​ ways and 3 other gentlemen from the remaining 6 in 6​C3​ ways. ∴ The number of ways in which both Mrs X and Mr Y are always included =7C2​×8​C3​=21×20=420 Hence the required number of committee in which Mrs X And Mr Y do not serve together= 1960-420 = 1540

Aakash Raavi

CMA Inter

★ 270

21-Mar-23 13:36

I asked question no 21?

Thread Starter

Virendra Saini

CA Inter

★ 0

21-Mar-23 13:37

Your solution for last question I know.

Thread Starter

Virendra Saini

CA Inter

★ 0

21-Mar-23 13:38

To reverse the decision, at least 5 out of the 9 judges must vote in favor of reversing the decision. Therefore, required number of ways = 9C5+9C6+9C7+9C8+9C9 =126+84+36+9+1 = 256

Aakash Raavi

CMA Inter

★ 270

21-Mar-23 13:43

Can you please understand in more simplicity?

Thread Starter

Virendra Saini

CA Inter

★ 0

21-Mar-23 13:46

I am understanding but not 100%? Means little bit different in understanding?

Thread Starter

Virendra Saini

CA Inter

★ 0

21-Mar-23 13:47

See this picture. You will get clarity

Aakash Raavi

CMA Inter

★ 270

21-Mar-23 13:50